NCERT Solutions for Class 9 Maths Chapter 6 - Lines And Angles

NCERT Solutions Class 9 Maths Chapter 6 – CBSE Term I Free PDF Download

NCERT Solutions for Class 9 Maths Chapter 6 Lines And Angles deals with the questions and answers related to the chapter Lines and Angles. This topic introduces you to basic Geometry primarily focusing on the properties of the angles formed i) when two lines intersect each other and ii) when a line intersects two or more parallel lines at distinct points. This chapter falls under the Unit – Geometry and is included for the Term I CBSE Syllabus of Class 9 Maths.

Now, you must be wondering why we are studying Lines and Angles. What are its real-life applications of it? The answer is that lines and angles are everywhere around us. The architecture uses lines and angles to design the structure of a building. When you stop at a signal and then move on when the signal light is green, then you either take a left angle turn or right-angle turn or move in a straight line. When you have to find the height of a tower or the location of an aircraft, then you need to know the angles. In NCERT Solutions for Class 9 Maths Chapter 6, you will learn to solve the questions related to all the concepts of Lines and Angles as per the updated term-wise CBSE syllabus for 2021-22. Students can avail a complete and free PDF of this chapter’s NCERT Solutions for Class 9 Maths from the link given below.

Download PDF of NCERT Solutions for Class 9 Maths Chapter 6 – Lines And Angles

Access Answers of Maths NCERT Class 9 Maths Chapter 6 – Lines And Angles

List of Exercises in class 9 Maths Chapter 6

Exercise 6.1 Solutions 6 Questions (5 Short Answer Questions, 1 Long Answer Question)
Exercise 6.2 Solutions 6 Questions (3 Short Answer Questions, 3 Long Answer Question)
Exercise 6.3 Solutions 6 Questions (5 Short Answer Questions, 1 Long Answer Question)

Class 9 Maths Chapter 6 Exercise: 6.1 (Page No: 96)

Exercise: 6.1 (Page No: 96)

1. In Fig. 6.13, lines AB and CD intersect at O. If AOC +BOE = 70° and BOD = 40°, find BOE and reflex COE.

Solution:

From the diagram, we have

(∠AOC +∠BOE +∠COE) and (∠COE +∠BOD +∠BOE) forms a straight line.

So, ∠AOC+∠BOE +∠COE = ∠COE +∠BOD+∠BOE = 180°

Now, by putting the values of ∠AOC + ∠BOE = 70° and ∠BOD = 40° we get

∠COE = 110° and ∠BOE = 30°

So, reflex ∠COE = 360^o – 110^o = 250^o

2. In Fig. 6.14, lines XY and MN intersect at O. If POY = 90° and a : b = 2 : 3, find c.

Solution:

We know that the sum of linear pair are always equal to 180°

So,

POY +a +b = 180°

Putting the value of POY = 90° (as given in the question) we get,

a+b = 90°

Now, it is given that a : b = 2 : 3 so,

Let a be 2x and b be 3x

∴ 2x+3x = 90°

Solving this we get

5x = 90°

So, x = 18°

∴ a = 2×18° = 36°

Similarly, b can be calculated and the value will be

b = 3×18° = 54°

From the diagram, b+c also forms a straight angle so,

b+c = 180°

c+54° = 180°

∴ c = 126°

3. In Fig. 6.15, PQR = PRQ, then prove that PQS = PRT.

Solution:

Since ST is a straight line so,

∠PQS+∠PQR = 180° (linear pair) and

∠PRT+∠PRQ = 180° (linear pair)

Now, ∠PQS + ∠PQR = ∠PRT+∠PRQ = 180°

Since ∠PQR =∠PRQ (as given in the question)

∠PQS = ∠PRT. (Hence proved).

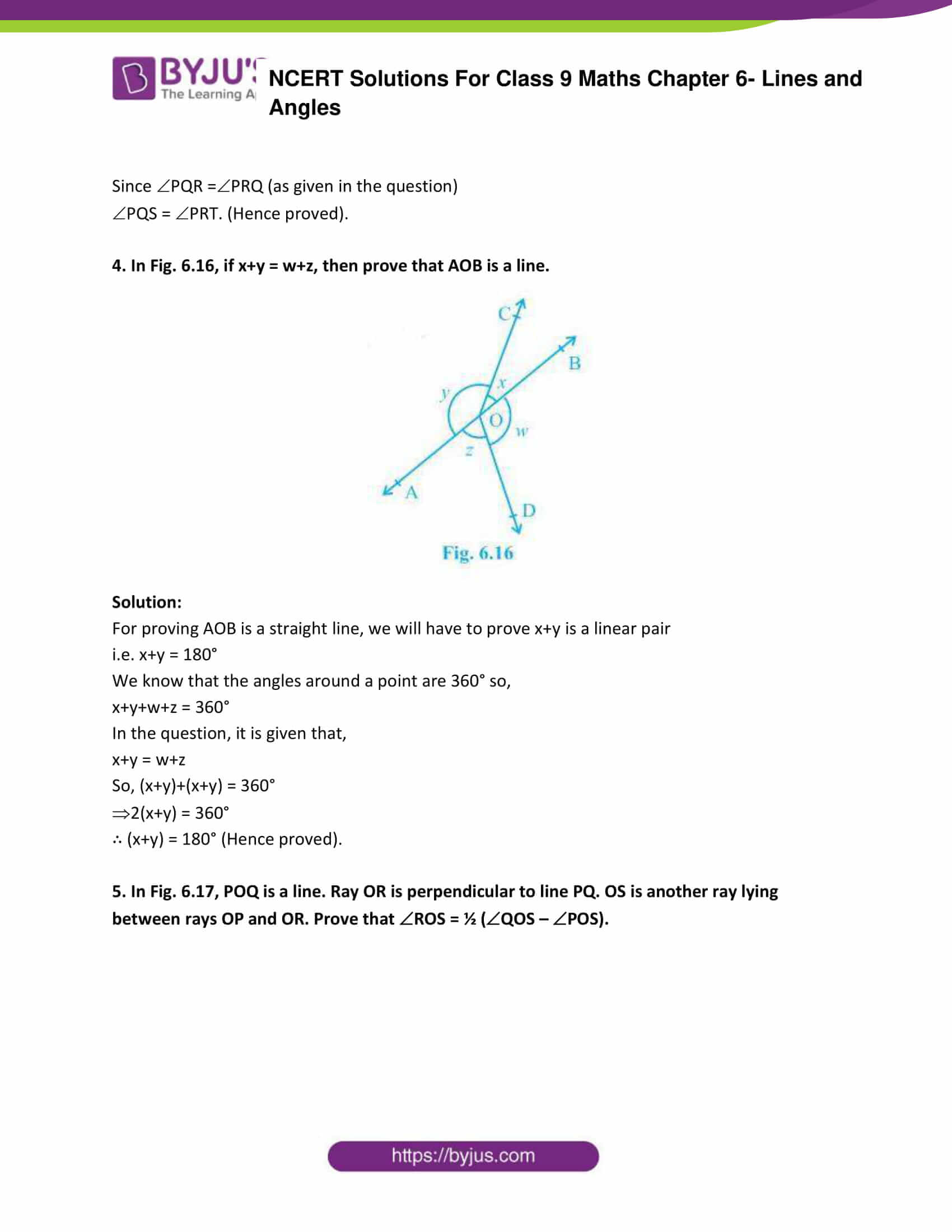
4. In Fig. 6.16, if x+y = w+z, then prove that AOB is a line.

Solution:

For proving AOB is a straight line, we will have to prove x+y is a linear pair

i.e. x+y = 180°

We know that the angles around a point are 360° so,

x+y+w+z = 360°

In the question, it is given that,

x+y = w+z

So, (x+y)+(x+y) = 360°

2(x+y) = 360°

∴ (x+y) = 180° (Hence proved).

5. In Fig. 6.17, POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that ROS = ½ (QOS – POS).

Solution:

In the question, it is given that (OR ⊥ PQ) and POQ = 180°

So, POS+ROS+ROQ = 180°

Now, POS+ROS = 180°- 90° (Since POR = ROQ = 90°)

∴ POS + ROS = 90°

Now, QOS = ROQ+ROS

It is given that ROQ = 90°,

∴ QOS = 90° +ROS

Or, QOS – ROS = 90°

As POS + ROS = 90° and QOS – ROS = 90°, we get

POS + ROS = QOS – ROS

2 ROS + POS = QOS

Or, ROS = ½ (QOS – POS) (Hence proved).

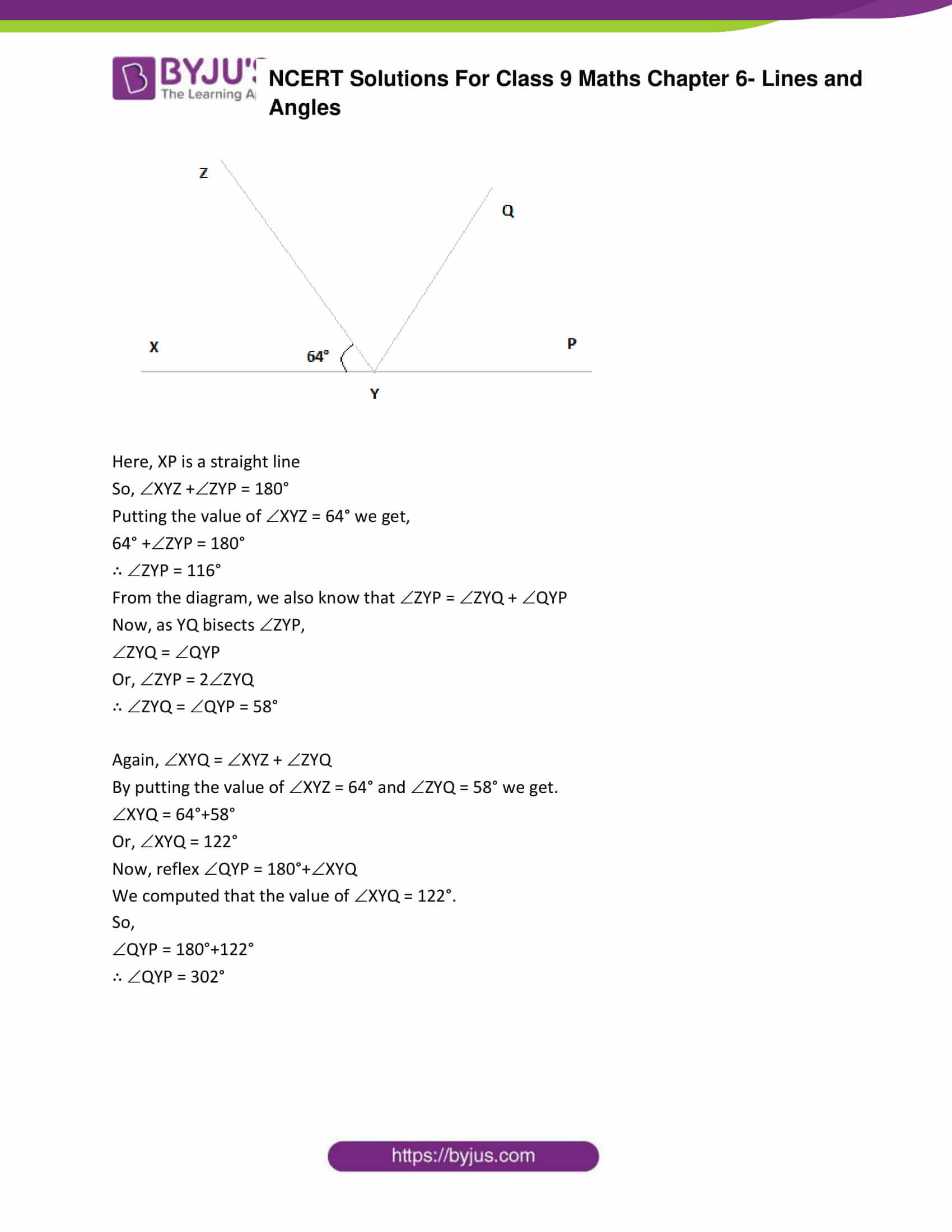
6. It is given that XYZ = 64° and XY is produced to point P. Draw a figure from the given information. If ray YQ bisects ZYP, find XYQ and reflex QYP.

Solution:

Here, XP is a straight line

So, XYZ +ZYP = 180°

Putting the value of XYZ = 64° we get,

64° +ZYP = 180°

∴ ZYP = 116°

From the diagram, we also know that ZYP = ZYQ + QYP

Now, as YQ bisects ZYP,

ZYQ = QYP

Or, ZYP = 2ZYQ

∴ ZYQ = QYP = 58°

Again, XYQ = XYZ + ZYQ

By putting the value of XYZ = 64° and ZYQ = 58° we get.

XYQ = 64°+58°

Or, XYQ = 122°

Now, reflex QYP = 180°+XYQ

We computed that the value of XYQ = 122°.

So,

QYP = 180°+122°

∴ QYP = 302°

Exercise: 6.2 (Page No: 103)

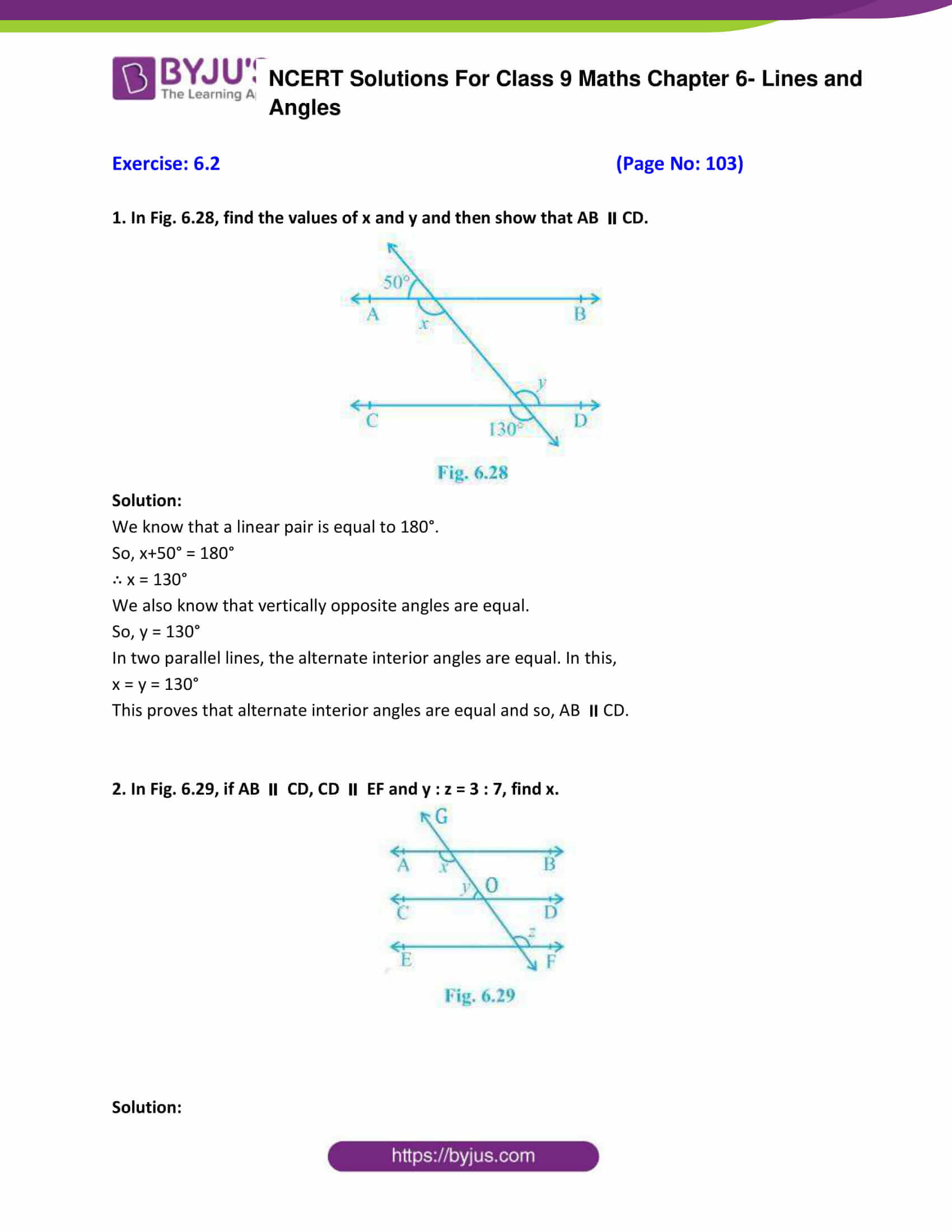
1. In Fig. 6.28, find the values of x and y and then show that AB CD.

Solution:

We know that a linear pair is equal to 180°.

So, x+50° = 180°

∴ x = 130°

We also know that vertically opposite angles are equal.

So, y = 130°

In two parallel lines, the alternate interior angles are equal. In this,

x = y = 130°

This proves that alternate interior angles are equal and so, AB CD.

2. In Fig. 6.29, if AB CD, CD EF and y : z = 3 : 7, find x.

Solution:

It is known that AB CD and CDEF

As the angles on the same side of a transversal line sums up to 180°,

x + y = 180° —–(i)

Also,

O = z (Since they are corresponding angles)

and, y +O = 180° (Since they are a linear pair)

So, y+z = 180°

Now, let y = 3w and hence, z = 7w (As y : z = 3 : 7)

∴ 3w+7w = 180°

Or, 10 w = 180°

So, w = 18°

Now, y = 3×18° = 54°

and, z = 7×18° = 126°

Now, angle x can be calculated from equation (i)

x+y = 180°

Or, x+54° = 180°

∴ x = 126°

3. In Fig. 6.30, if AB CD, EF ⊥ CD and GED = 126°, find AGE, GEF and FGE.

Solution:

Since AB CD, GE is a transversal.

It is given that GED = 126°

So, GED = AGE = 126° (As they are alternate interior angles)

Also,

GED = GEF +FED

As EF⊥ CD, FED = 90°

∴ GED = GEF+90°

Or, GEF = 126° – 90° = 36°

Again, FGE +GED = 180° (Transversal)

Putting the value of GED = 126° we get,

FGE = 54°

So,

AGE = 126°

GEF = 36° and

FGE = 54°

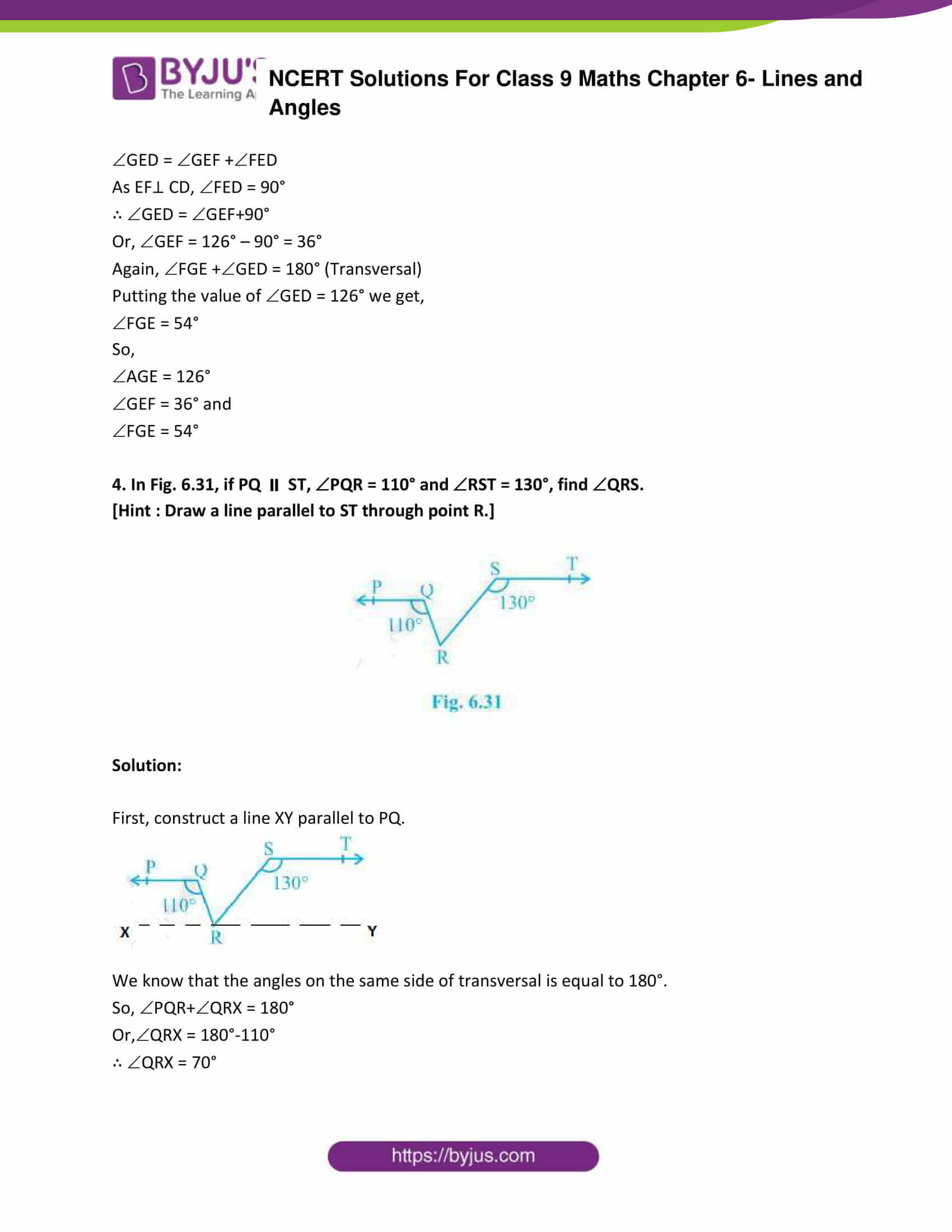
4. In Fig. 6.31, if PQ ST, PQR = 110° and RST = 130°, find QRS.

[Hint : Draw a line parallel to ST through point R.]

Solution:

First, construct a line XY parallel to PQ.

We know that the angles on the same side of transversal is equal to 180°.

So, PQR+QRX = 180°

Or,QRX = 180°-110°

∴ QRX = 70°

Similarly,

RST +SRY = 180°

Or, SRY = 180°- 130°

∴ SRY = 50°

Now, for the linear pairs on the line XY-

QRX+QRS+SRY = 180°

Putting their respective values, we get,

QRS = 180° – 70° – 50°

Hence, QRS = 60°

5. In Fig. 6.32, if AB CD, APQ = 50° and PRD = 127°, find x and y.

Solution:

From the diagram,

APQ = PQR (Alternate interior angles)

Now, putting the value of APQ = 50° and PQR = x we get,

x = 50°

Also,

APR = PRD (Alternate interior angles)

Or, APR = 127° (As it is given that PRD = 127°)

We know that

APR = APQ+QPR

Now, putting values of QPR = y and APR = 127° we get,

127° = 50°+ y

Or, y = 77°

Thus, the values of x and y are calculated as:

x = 50° and y = 77°

6. In Fig. 6.33, PQ and RS are two mirrors placed parallel to each other. An incident ray AB strikes the mirror PQ at B, the reflected ray moves along the path BC and strikes the mirror RS at C and again reflects back along CD. Prove that AB CD.

Solution:

First, draw two lines BE and CF such that BE ⊥ PQ and CF ⊥ RS.

Now, since PQ RS,

So, BE CF

We know that,

Angle of incidence = Angle of reflection (By the law of reflection)

So,

1 = 2 and

3 = 4

We also know that alternate interior angles are equal. Here, BE ⊥ CF and the transversal line BC cuts them at B and C

So, 2 = 3 (As they are alternate interior angles)

Now, 1 +2 = 3 +4

Or, ABC = DCB

So, AB CD alternate interior angles are equal)

Exercise: 6.3 (Page No: 107)

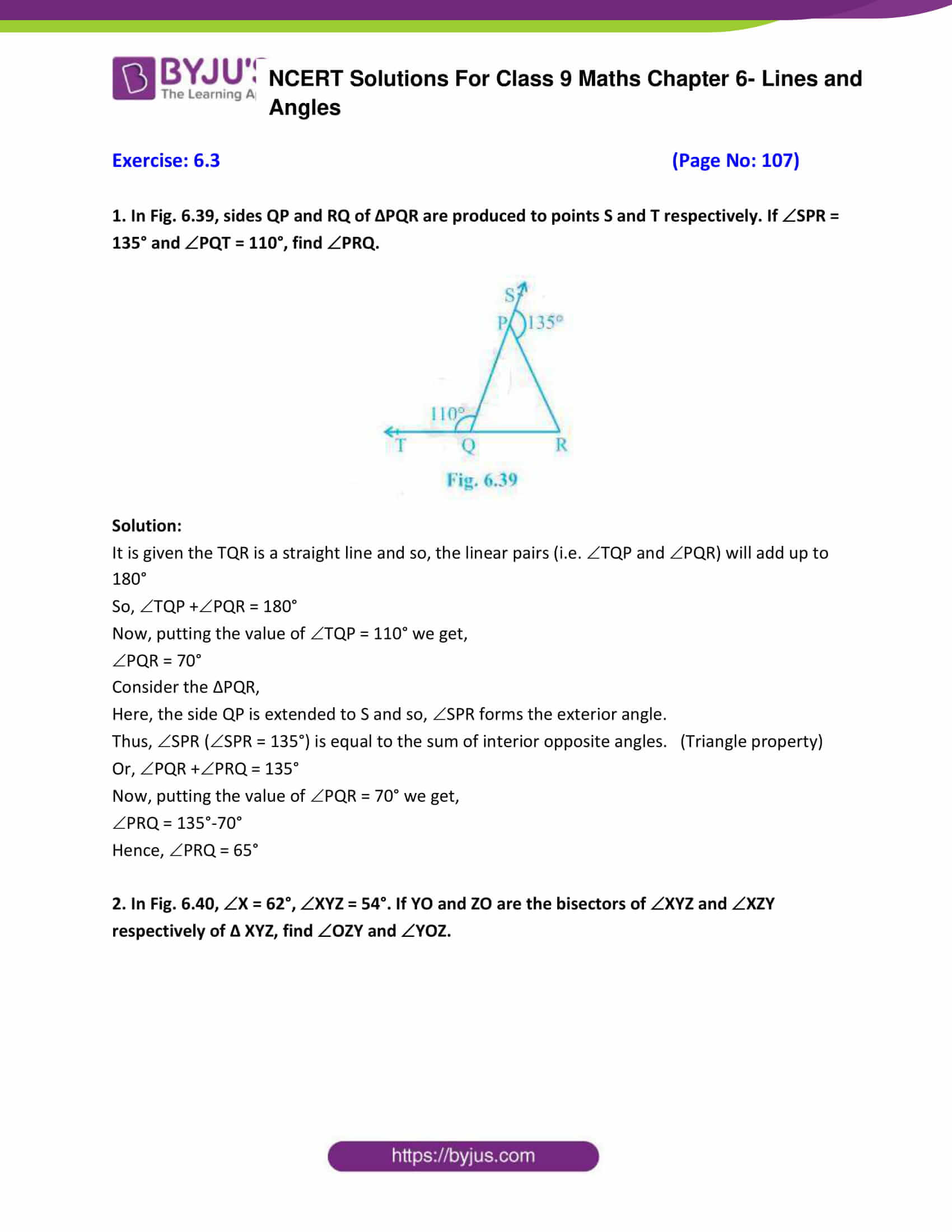
1. In Fig. 6.39, sides QP and RQ of ΔPQR are produced to points S and T respectively. If SPR = 135° and PQT = 110°, find PRQ.

Solution:

It is given the TQR is a straight line and so, the linear pairs (i.e. TQP and PQR) will add up to 180°

So, TQP +PQR = 180°

Now, putting the value of TQP = 110° we get,

PQR = 70°

Consider the ΔPQR,

Here, the side QP is extended to S and so, SPR forms the exterior angle.

Thus, SPR (SPR = 135°) is equal to the sum of interior opposite angles. (Triangle property)

Or, PQR +PRQ = 135°

Now, putting the value of PQR = 70° we get,

PRQ = 135°-70°

Hence, PRQ = 65°

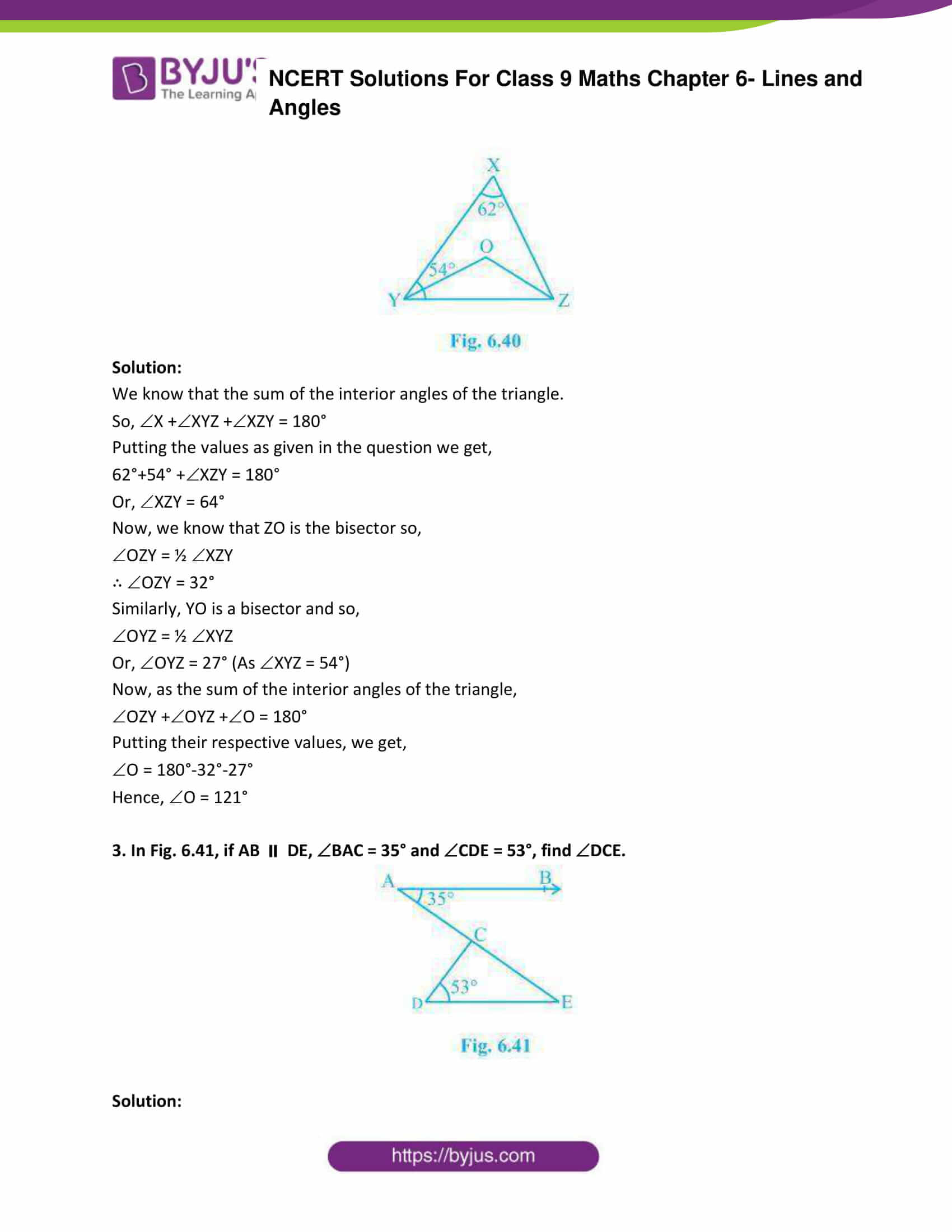
2. In Fig. 6.40, X = 62°, XYZ = 54°. If YO and ZO are the bisectors of XYZ and XZY respectively of Δ XYZ, find OZY and YOZ.

Solution:

We know that the sum of the interior angles of the triangle.

So, X +XYZ +XZY = 180°

Putting the values as given in the question we get,

62°+54° +XZY = 180°

Or, XZY = 64°

Now, we know that ZO is the bisector so,

OZY = ½ XZY

∴ OZY = 32°

Similarly, YO is a bisector and so,

OYZ = ½ XYZ

Or, OYZ = 27° (As XYZ = 54°)

Now, as the sum of the interior angles of the triangle,

OZY +OYZ +O = 180°

Putting their respective values, we get,

O = 180°-32°-27°

Hence, O = 121°

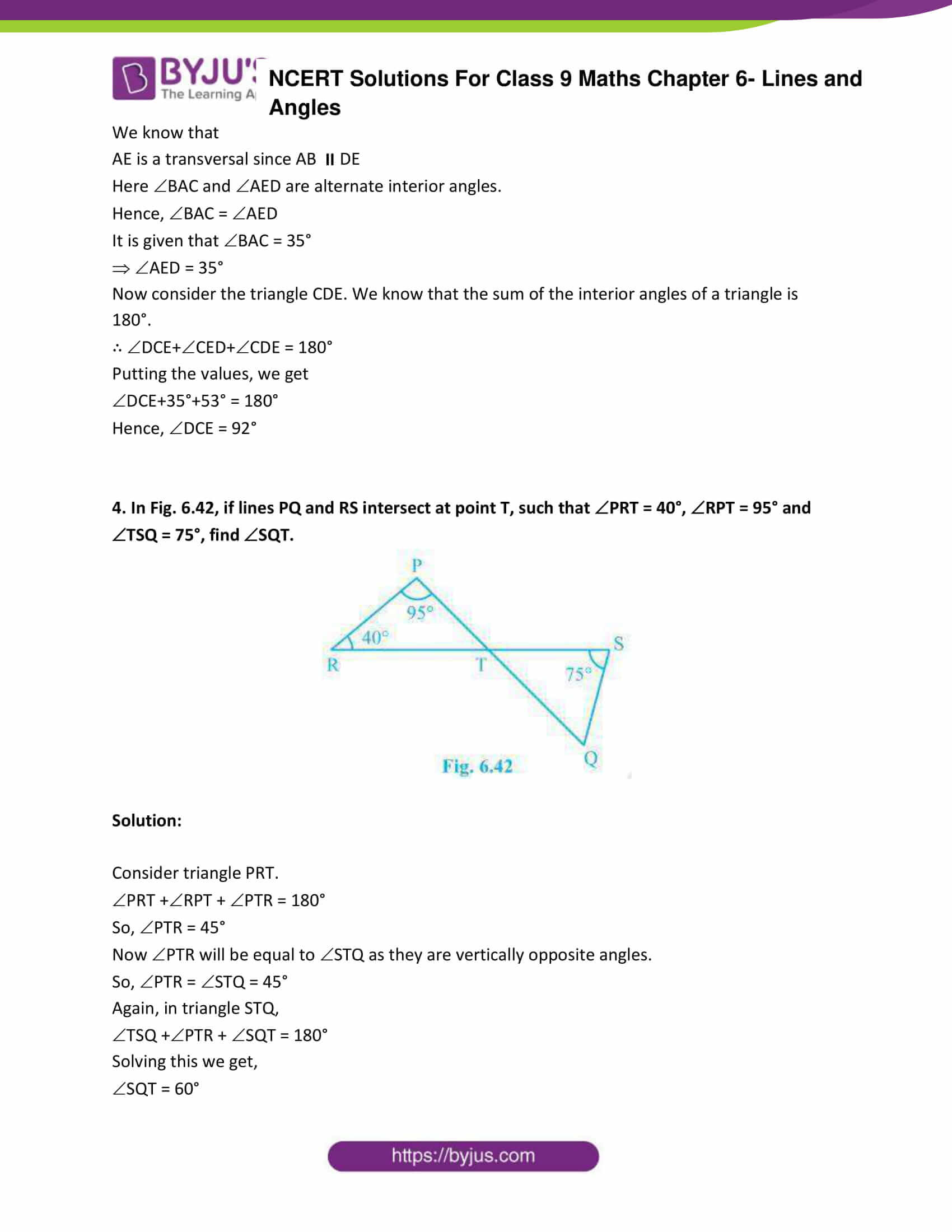
3. In Fig. 6.41, if AB DE, BAC = 35° and CDE = 53°, find DCE.

Solution:

We know that AE is a transversal since AB DE

Here BAC and AED are alternate interior angles.

Hence, BAC = AED

It is given that BAC = 35°

AED = 35°

Now consider the triangle CDE. We know that the sum of the interior angles of a triangle is 180°.

∴ DCE+CED+CDE = 180°

Putting the values, we get

DCE+35°+53° = 180°

Hence, DCE = 92°

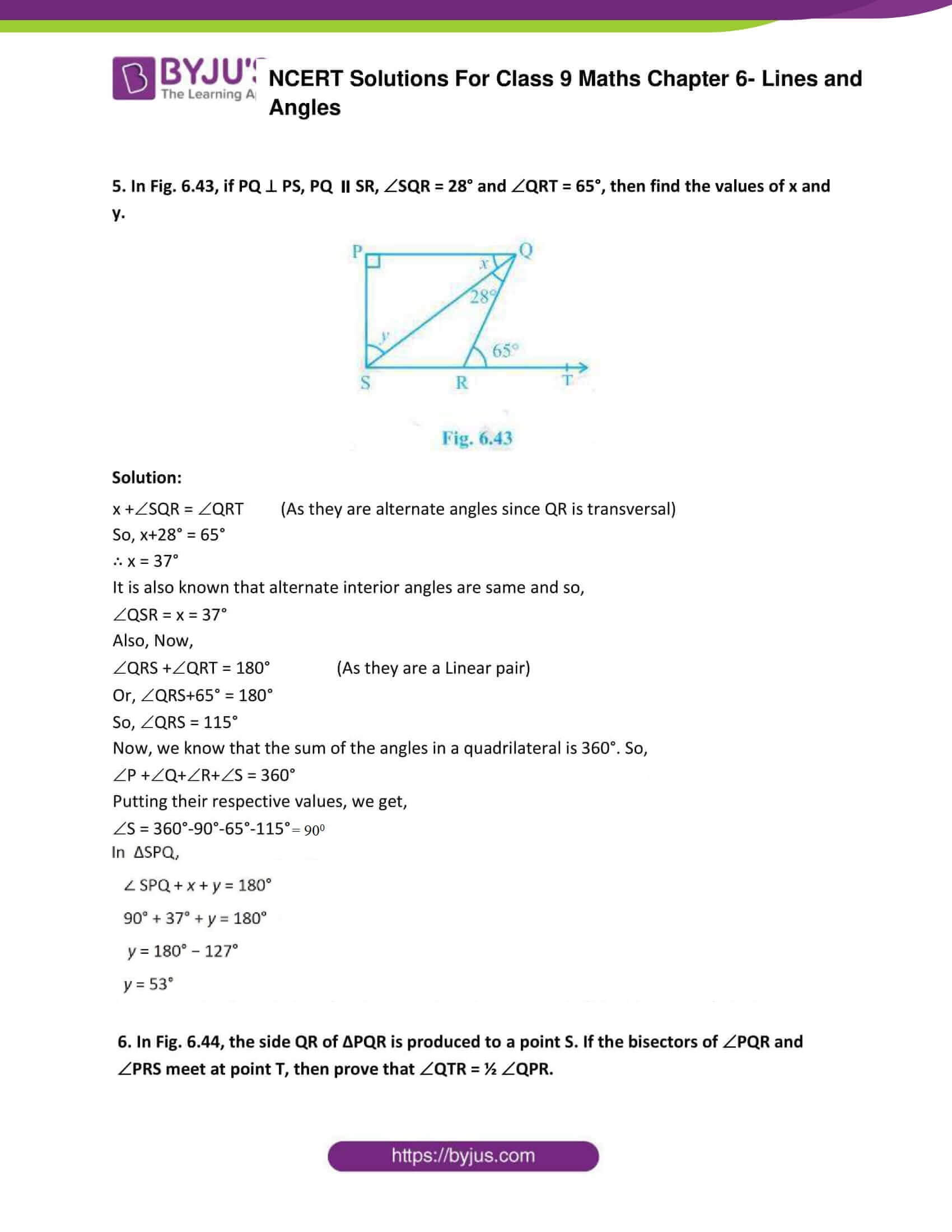
4. In Fig. 6.42, if lines PQ and RS intersect at point T, such that PRT = 40°, RPT = 95° and TSQ = 75°, find SQT.

Solution:

Consider triangle PRT.

PRT +RPT + PTR = 180°

So, PTR = 45°

Now PTR will be equal to STQ as they are vertically opposite angles.

So, PTR = STQ = 45°

Again, in triangle STQ,

TSQ +PTR + SQT = 180°

Solving this we get,

SQT = 60°

5. In Fig. 6.43, if PQ ⊥ PS, PQ SR, SQR = 28° and QRT = 65°, then find the values of x and y.

Solution:

x +SQR = QRT (As they are alternate angles since QR is transversal)

So, x+28° = 65°

∴ x = 37°

It is also known that alternate interior angles are same and so,

QSR = x = 37°

Also, Now,

QRS +QRT = 180° (As they are a Linear pair)

Or, QRS+65° = 180°

So, QRS = 115°

Now, we know that the sum of the angles in a quadrilateral is 360°. So,

P +Q+R+S = 360°

Putting their respective values, we get,

S = 360°-90°-65°-115°

In Δ SPQ

∠SPQ + x + y = 180⁰

90⁰ + 37⁰ + y = 180⁰

y = 180⁰ – 127⁰ = 53⁰

Hence, y = 53°

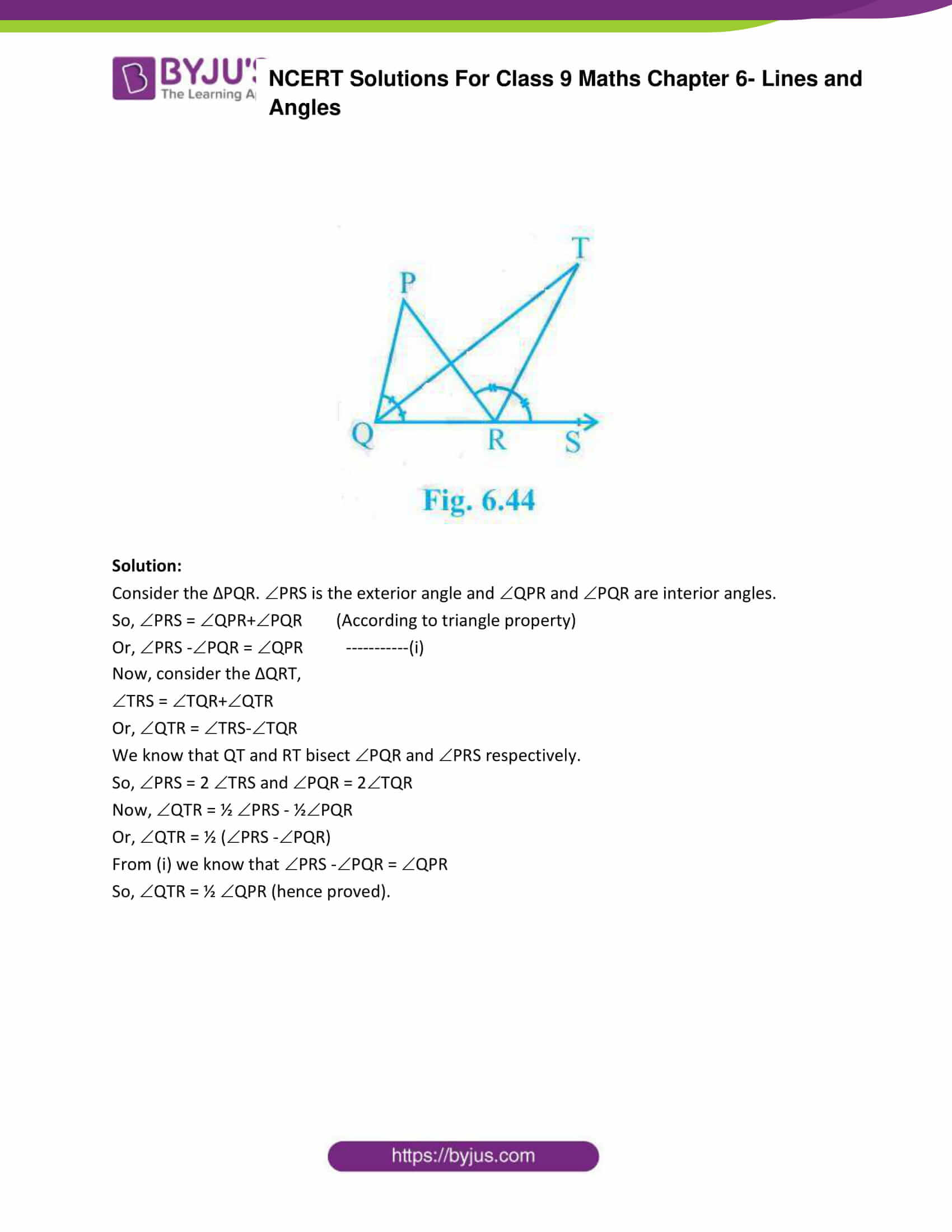
6. In Fig. 6.44, the side QR of ΔPQR is produced to a point S. If the bisectors of PQR and PRS meet at point T, then prove that QTR = ½ QPR.

Solution:

Consider the ΔPQR. PRS is the exterior angle and QPR and PQR are interior angles.

So, PRS = QPR+PQR (According to triangle property)

Or, PRS -PQR = QPR ———–(i)

Now, consider the ΔQRT,

TRS = TQR+QTR

Or, QTR = TRS-TQR

We know that QT and RT bisect PQR and PRS respectively.

So, PRS = 2 TRS and PQR = 2TQR

Now, QTR = ½ PRS – ½PQR

Or, QTR = ½ (PRS -PQR)

From (i) we know that PRS -PQR = QPR

So, QTR = ½ QPR (hence proved).

NCERT Solutions for Class 9 Maths Chapter 6 – Lines And Angles

The Class 9 Maths theory paper is of 80 marks. Out of which Geometry constitute a total of 22 marks which includes Introduction to Euclid’s Geometry, Lines and Angles, Triangles, Quadrilaterals, Areas, Circles, Constructions. As you can see that it constitutes approximately 27% of weightage. So, using the NCERT Solutions for Class 9, students can easily score high marks if by having a thorough understanding of this topic.

Solve all the exercise problems of Lines and Angles. Refer to the NCERT Solutions of Class 9 provided by our Experts below. It will help you to solve the questions in an easy way.

Summary of NCERT Solutions for Class 9 Maths Chapter 6 Lines And Angles

Before starting to solve the exercise problems, you must first read the theory part and get to know the basic terms, definitions and theorems. After that go through the solved examples of Lines and Angles that are given in the Class 9 NCERT Book. It will make your concepts more clear. Then you can start solving the exercise problems with the help of NCERT Solutions.

Key Points of NCERT Class 9 Maths Chapter 6 – Lines And Angles

After solving the Line and Angles chapter of Class 9 Maths, you will get to know the following points:

1. Basic terms and definitions related to a line segment, ray, collinear points, non-collinear points, intersecting and non-intersecting lines.
2. Pair of angles (reflex, complementary, supplementary, adjacent, vertical opposite, linear pair).
3. Parallel and Transversal Lines and theorems related to them.
4. Angle sum property of a triangle.

We hope this information on “NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles” is useful for students. Stay tuned for further updates on CBSE and other competitive exams. To access interactive Maths and Science Videos download BYJU’S App and subscribe to YouTube Channel.

To learn more concepts regarding Lines and Angles, students can access the other study materials which are prescribed by the CBSE board. By doing this, students will be able to solve the questions that would appear in the CBSE Term I exams.

• RD Sharma Solutions for Class 9 Maths Chapter 8 Lines and Angles